
 Home Home |
 For authors For authors |
 Submission status Submission status |
 Archive Archive
|
 Archive
(English) Archive
(English)
|
 Current Current
|
 Volumes 93-112 Volumes 93-112 |
 Volumes 113-119 Volumes 113-119 |
| Volume 119 |
| Volume 118 |
| Volume 117 |
| Volume 116 |
| Volume 115 |
| Volume 114 |
| Volume 113 |
 Search Search |
| VOLUME 113 (2021) | ISSUE 11 | PAGE 729 |
|---|
|
Aharonov-Bohm interferometry based on helical edge states1) (Mini-review)
R. A. Niyazov+* 2), D. N. Aristov+*, V. Yu. Kachorovskii×
+Department of Physics, St. Petersburg State University, 198504 St. Petersburg, Russia *National Research Centre "Kurchatov Institute", Petersburg Nuclear Physics Institute, 188300 Gatchina, Russia ×Ioffe Institute, 194021 St. Petersburg, Russia
Abstract
We review recent studies of the spin-dependent tunneling transport via Aharonov-Bohm interferometer (ABI) formed by helical edge states. We focus on the experimentally relevant case of relatively high temperature, T, as compared to level spacing, Δ. The tunneling conductance of helical ABI is structureless in ballistic case but shows sharp periodic antiresonances as a function of magnetic flux - with the period hc/2e - in presence of magnetic impurities. The incoming unpolarized electron beam acquires finite polarization after transmission through the helical ABI provided that the edges contain at least one magnetic impurity. The finite polarization appears even in the fully classical regime and is therefore robust to dephasing. There is also a quantum contribution to the polarization, which shows sharp identical resonances as a function of magnetic flux with the same period as conductance. This polarization survives at relatively high temperature. The interferometer can be described in terms of ensemble of 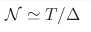 flux-tunable qubits
giving equal contributions to conductance and spin polarization. Hence,
with increasing the temperature number of active qubits participating in
the charge and spin transport increases.
These features of tunneling helical ABI open a wide avenue for
applications in the area of quantum computing. flux-tunable qubits
giving equal contributions to conductance and spin polarization. Hence,
with increasing the temperature number of active qubits participating in
the charge and spin transport increases.
These features of tunneling helical ABI open a wide avenue for
applications in the area of quantum computing.
|